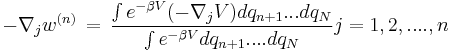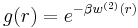Potential of mean force

The Potential of Mean Force [1] of a system with N molecules is strictly the potential that gives the average force over all the configurations of all the n+1...N molecules acting on a particle at any fixed configuration keeping fixed a set of molecules 1...n

-\nabla_jw^{(n)} \, = \, \frac {\int e^{-\beta V} (- \nabla_j V)d q_{n%2B1}...dq_N } {\int e^{-\beta V} d q_{n%2B1} ....dq_N} j =1,2,....,n

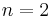For n=2 , w^{(2)}(r) is the average work needed to bring the two particles from infinite separation to a distance r . It is also related to the radial distribution function of the system, g(r) , by[2]:

g(r) = e^{-\beta w^{(2)}(r)}

Contents

See also

References

  1. ^ Kirkwood, J. G. Statistical Mechanics of fluid Mixtures. J. Chem. Phys. 1935, 3, 300; Statistical Mechanics of Liquid Solutions. Chemical Reviews 1936, 19, 275.
  2. ^ See Chandler, section 7.3

Further reading

External links